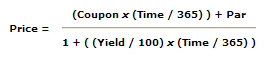| Every so often there will come a time when the PCs have a little extra cash burning a hole in their pockets, and rather than spend it all they'll want to invest it for a rainy day. Many of the worlds of the Imperium have some sort of capitalist economy and will provide a variety of local investment options, but such investments will be in the local economy and therefore at risk of local economic conditions, exchange rate fluctuations, planetary tax laws, and even nationalisation (if the local political mood turns that way). This is where the Interstellar Financial Market System comes in. Under the regulation of the Ministry of Commerce each Imperial subsector capitol has an Interstellar Financial Market (IFM). An IFM is a 'place' to publicly trade financial instruments denominated in Imperial Credits (Crimps). There are a variety of instrument types, each with their own risks and returns, but at the end of the day the choice of instrument is a matter of personal preference. This document covers basic investment instrument types only, and does not cover convertible bonds, preferred shares, deferred shares, unit trusts, strips, STIFs, repos, currency exchanges, spot rates, forward rates, hedging, futures, swaps, options, swoptions, margin calls, ... etc. (Maybe I'll add these later.) One of the problems with a stock market in Traveller is the time lag issue. However, given that a subsector stock market will be situated on a high TL planet any attempt to artificially slow down trading will give rise to grey markets: financial institutions on the planet will start trading directly with each other and bypass any imposed restrictions. Thus no such restrictions are imposed, and it is important for offworld investors to have a specific client mandate with their stockbroker. (A client mandate is a set of instructions on what to do in the investor's absence. For example, it could include "sell any shares whose price drops below par value, use surplus funds to buy shares in manufacturers of environmentally friendly products".) Another problem is the negative effect of institutional investors (usually large pension funds) on corporate ethics. Most capitalist planets have laws requiring pension fund managers to maximise the value of their holdings taking into account only the risk of an investment defaulting on its payments. Where institutions under such restrictions are major investors corporations are under pressure to pursue profit without regard for ethical considerations. This in turn can give rise to anti-capitalist sentiment. The Ministry of Commerce, therefore, has numerous regulations and guidelines in place to restrict this. Thus there is a higher percentage of private investors to institutional investors in the IFM than in many local planetary exchanges. Stockholders can also be held liable for corporate misbehavior "on their behalf". Every buy or sell on the IFM is subject to broker's fees and stamp duty (placing and cashing a simple deposit, or creating a CD are exempt). Broker's fees are 1/1000th of the cash value of the transaction. Stamp duty (named after the archane practice where all transaction receipts had to be stamped by a witnessing official) is payable to the IFM service and is a flat Cr0.50 per transaction regardless of value. The IFM also acts as a Custodian. That is, rather than constantly moving millions of stock certificates every time a transaction takes place, almost all of these certificates are kept in the IFM's ultra-high security vaults. An electronic register of actual ownership is then merely updated instead. On the extremely rare occasions that certificates are required it usually takes 14 days after a transaction for the certificates to be delivered. This stock cannot be traded until being redeposited with the custodian. |
| Every IFM has one or more investment services that grade instruments according to their risk of defaulting on scheduled payments (dividends, coupons, and/or principle repayments). By convention these grades take the form of 10 standardised codes, though there are sometimes disagreements between investment services as to what grade a specific instrument might get.
Due to the communications lag offworld investors are strongly advised to avoid instruments with a "non-investment" grade. Companies that have filed a petition of bankruptcy or who are currently defaulting on payments are not permitted to take on new business, though their existing instruments may still be traded. | ||||||||||||||||||||||||
| The simplest of all market instruments is cash itself. The cash is simply deposited with a bank with a commitment not to withdraw it until a set date (called the maturity date). When the deposit matures interest is paid. Interest is computed on a simple basis (ie. no compounding), and rates are sometimes referred to in base points (where 100 base points = 1%). Deposits cannot be 'traded' in the normal sense of the word. Interest rates will vary based on grade and duration (from deposit to maturity).
For intermediate durations use the lower rate. The actual interest rate offered by a specific bank on a specific day will vary by (2d6-7 x 5) base points. If the PCs negotiate then add their Broker skill to the roll. For example: Deposits are made in units of Cr10,000. So if the PCs have Cr15,000 they could place Cr10,000 in a deposit account and negotiate a maturity date and rate, the other Cr5,000 could be placed in an ordinary current account (earning no interest). Amounts withdrawn before the agreed maturity date do not earn interest. So if the PCs were to place their Cr10,000 in a 1 year deposit account but then withdraw it after 360 days they would gain no interest. You might expect that a simple deposit would have a AAA grade but banks can and do lend out 90% of all deposits ... if they make too many unwise loans they can go bankrupt and the PCs will be lucky to get any of their deposit back. Therefore the prudent investor will consider the grade of the bank before making a deposit. [In the rare event that a bank collapses it will take 1d6 / 2 years before any of a deposit can be recovered, and the recovered amount will only by 2d6 percent. If someone has a loan from a bank that collapses they can expect strong demands for immediate repayment by the bank's liquidator and may need a lawyer to prevent seizure of assets. There is, however, a 1 in 6 chance that another bank will buy the collapsed bank (for Cr1) and honour all its debts ... if it thinks there were exceptional circumstances and it wants the fallen bank's customer base and talent.] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Banks would like to be able to take deposits from customers on the understanding that these deposits would not be repayable in the short term. However, generally investors are either unwilling to commit their funds for specified time periods or demand too high a premium. The solution is the creation of certificates of deposit (CDs). CDs carry a fixed annual coupon (annual interest paid on the deposit) and usually have a maturity of at least five years. These certificates can be traded enabling the deposit holder to get their deposit back through the sales proceeds and not withdrawal. (In effect one investor's deposit is replaced with another investor's deposit seamlessly.)
For intermediate durations use the lower rate. As with ordinary deposits when setting up a CD the actual interest rate offered by a specific bank on a specific day will vary by (2d6-7 x 5) base points. If the PCs negotiate then add their Broker skill to the roll. For example: CDs are made in units of Cr10,000. So if the PCs have Cr15,000 they could create a Cr10,000 CD with a negotiated maturity date and rate, the other Cr5,000 could be placed in an ordinary current account (earning no interest). CDs cannot be withdrawn, but they can be traded. In simple terms the value the CD will sell for is based on how much money the CD will generate (including the principle) and how long the new investor will have to wait for his money. The term Stated Yield is just another way of saying interest rate. The Required Yield is what the market thinks the rate should be and it fluctuates ... generally it is 1d6 x 10 base points below the Stated Yield. Subtract broker skill from roll, if roll is zero or less then use 2d6 base points. For example: However, IFM CDs usually have multiple year maturities and so the calculation is complicated by the fact that some of the money will be paid 'early' (ie. the remaining coupons). So recalculate each year starting with the last and working back. For example: (See deposit section for notes on bank collapse.) | ||||||||||||||||||||||||||||||||||||||||||||||||||
| A producer may sell goods to an offworld consumer. The producer will, however, be unwilling to release the goods without some guarantee of payment. This payment usually takes the form of a Bill of Exchange drawn by the offworld importer promising to pay an agreed sum within a set period, up to 120 days. (A Bill of Exchange is a promissory note ... in effect a post-dated cheque ... which commits the issuer to pay a specified sum on a specified date in the future.) In itself this would be regarded as inadequate since there would still be the risk of default. Therefore merchant banks with branches in both producer's and importer's systems will extend a guarantee facility ... they will undertake to honour the Bill in the event of default by the importer. The megacorporations and many of the larger LICs have realised that their own names were strong enough to enable them to issue bills without the need for a bank guarantee. These special bills are called Commercial Paper, which are simply the promissory notes issued at a discount to the face value with maturities normally up to 12 months. Commercial Paper can be traded to third parties on an IFM. (As the time to the maturity date decreases the value increases from its original discounted value to its face value.) Regulations stipulate that Commercial Paper must be backed by a specific trade and thus cannot be issued as a general money raising method.
As with ordinary deposits the actual interest rate of commercial paper offered by a specific corporation on a specific day will vary by (2d6-7 x 10) base points. Commercial Paper calculations are similar to CD calculations, yet simpler. There are three differences:
For example: | ||||||||||||||||||
| "In general terms a bond is a negotiable debt instrument issued by a borrower for a fixed period of time paying interest known as the coupon, which is fixed at the issue date and is paid regularly to the holder of the bond until it is redeemed at maturity when the principle amount is repaid." In other words bonds are glorified IOUs. They can be traded on the IFM on which they are issued, the face value of a bond is paid out to the holder when it matures, and there are intermediate payments (usually annually) to the current holder. While corporations may raise funds (for example to redevelop a factory) by issuing bonds in local planetary markets (where permitted under local laws and conditions), offworld bonds must be issued through the local IFM. In addition to corporations needing to raise funds through the issue of bonds, so too do governments. Bonds issued by the Imperium and by sector and subsector governments are termed Gilts. The term comes from the fact that on the rare occasions that an actual physical certificate of ownership of a gilt is issued the certificate has a gold (or gilt) edge ... thus they are gilt-edged bonds (aka Gilts). Since the risk of defaulting is so low as to be practically non-existent gilts are considered totally safe but have a correspondingly low return ... they form the interest rate baseline. (Corporate bonds, also called debentures, carry a larger risk and thus pay a larger return in compensation.)
As with ordinary deposits the actual interest rate of debentures offered by a specific corporation on a specific day will vary by (2d6-7 x 10) base points from the gilt baseline. All gilts, on the other hand, vary by (2d6-7 x 10) base points on a monthly basis (and remain fixed for the month) ... so for debentures apply both the debenture's specific daily variation and the general monthly gilt variation. The basic yield of a bond is the annual coupon divided by the market price, but as bonds can be sold at other than face value (aka Par) the following calculation is made:
If general interest rates go up then bonds don't look so favourable in comparison with other investment instruments and so people will sell. This drives the price down which in turn increase the yield. Once the yield matches the new interest rate things stabilise. If interest rates go down the same process works in reverse. Thus the price will alter to keep the yield tracking the interest rates. The above formula can be rewritten thus:
By convention all bonds have a par value of Cr100, therefore both original cost and current market price is expressed as for Cr100 of bond. For example: | ||||||||||||||||||||||||||||||
| It is also possible to own some or all or a corporation. If a company is created with an initial investment of MCr1 (for example) this might be defined as 100,000 shares each worth Cr10. Some of that initial investment may be raised by selling some of the shares in the market. As the company makes profit that profit is distributed to the shareholders in equal amounts per share (called a dividend), though some may be held back for reinvestment in the company. Sometimes an existing company may wish to raise additional investment ... and so will create additional shares to sell on the market.
The par value for a typical stock is [BP]d6 x Cr10. For this simulation use the par value as the starting price (this can be made more accurate by rolling 2d6-2 for each digit ... 6 decimal places are kept for possible large holdings). For example: Each week the price will vary by ... ( 2d6 / 1d6 ) x Price x Price Mod. To determine the direction of change assume that week 0 was an upward trend. Then each week roll 2d6 ... if the roll is 11+ reverse the trend. Modify this roll by adding the number of weeks since the last trend reversal. For example: But share price changes are not where you make money (unless you are a short-term rapid speculator). Each year the profits of a corporation are divided up amongst the shareholders. The portion of profit per share is called the dividend. Pick a day of the year for the corporation's annual dividend payment date (local planetary stock markets typically have quarterly dividend payments but IFM stocks are annually based to allow offworld reports to arrive). Regardless of what's rolled the price trend will always be upward in the preceding 4 weeks before a dividend payment, and will always be downward in the week after a dividend payment. The size of the annual dividend is ... Dividend = Par x Dividend Mult x (1d6 - Dividend Mod) If a zero dividend is declared then multiply the next week's automatic price drop by 1d6. If this roll is a 6 then also drop the share's grade by 1. However, if the dice roll in the dividend calculation is 6 then increase the share's grade by 1 (max = AA). If a grade C share declares a zero dividend then it automatically becomes grade D. For example: Sometimes a desirable share will have a price that's too high to be affordable to smaller investors. The solution is a split: each share is replaced by 2 new shares that have half the par value and half the price. Except in the 5 weeks around a dividend payment date anytime a share is trading at more than double its par value roll 1d6 ... on a 6 the share splits. Most small and medium sized LICs will be registered (ie. their shares are traded) with only one IFM. This will be the IFM nearest their corporate headquarters. But this in unfeasible for large LICs and megacorporations. The common solution is to organise large corporations into regional operating divisions, each with its own class of shares (registered on the appropriate IFM). If one region performs poorly it must be proped up by fresh capital from nearby regions ... thus having a ripple effect throughout the corporation. In the case of megacorporations they are also organised into functional branches as well. For example: | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||